Tellusant’s Universal Profit Equation (TUPE)
- 2024-03-21
- 11:55 am
- Posted By: Tellusant
Pricing optimization is the fastest way to increase profits. Here we describe methods for how companies can achieve this.
These methods are universal. We here go into depth describing pricing within consumer goods.
In the following we discuss:
- The Tellusant universal profit equation
- Pricing approach in consumer goods
- Conclusions
TELLUSANT UNIVERSAL PROFIT EQUATION
Every company follows the basic equation: profit = revenue − cost. Tellusant has extended it to be more actionable, what we call the Tellusant Universal Profit Equation (TUPE).
It uses one revenue component and three cost components as shown in the equation below.
Executives are used to thinking about variable and fixed costs. Here we explicitly break out discretionary costs that are neither variable nor fixed but chosen at the discretion of management.
The beauty of TUPE is that:
- It is parsimonious (exactly what is required — nothing more, nothing less) and applies to all industries
- It breaks out discretionary costs that a company can choose to incur, or not
- It lends itself to more detailed expansions with elasticities and other commonly used concepts
With this as the starting point, we now turn to pricing in consumer goods. We leverage a brilliant paper by Sethuraman and Tellis throughout.¹
PROFIT OPTIMIZATION IN CONSUMER GOODS
TUPE adapted to consumer goods is shown below. It includes advertising/promotion and assumes that R&D and other discretionary costs are low.
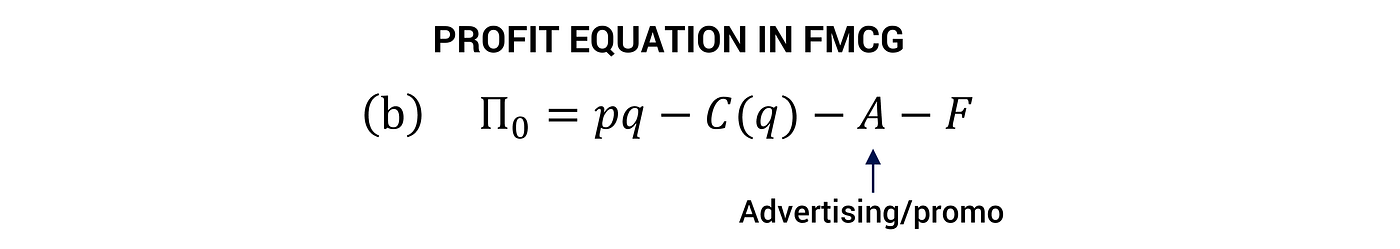
Pricing
To make the equation come alive, we introduce three ratios:
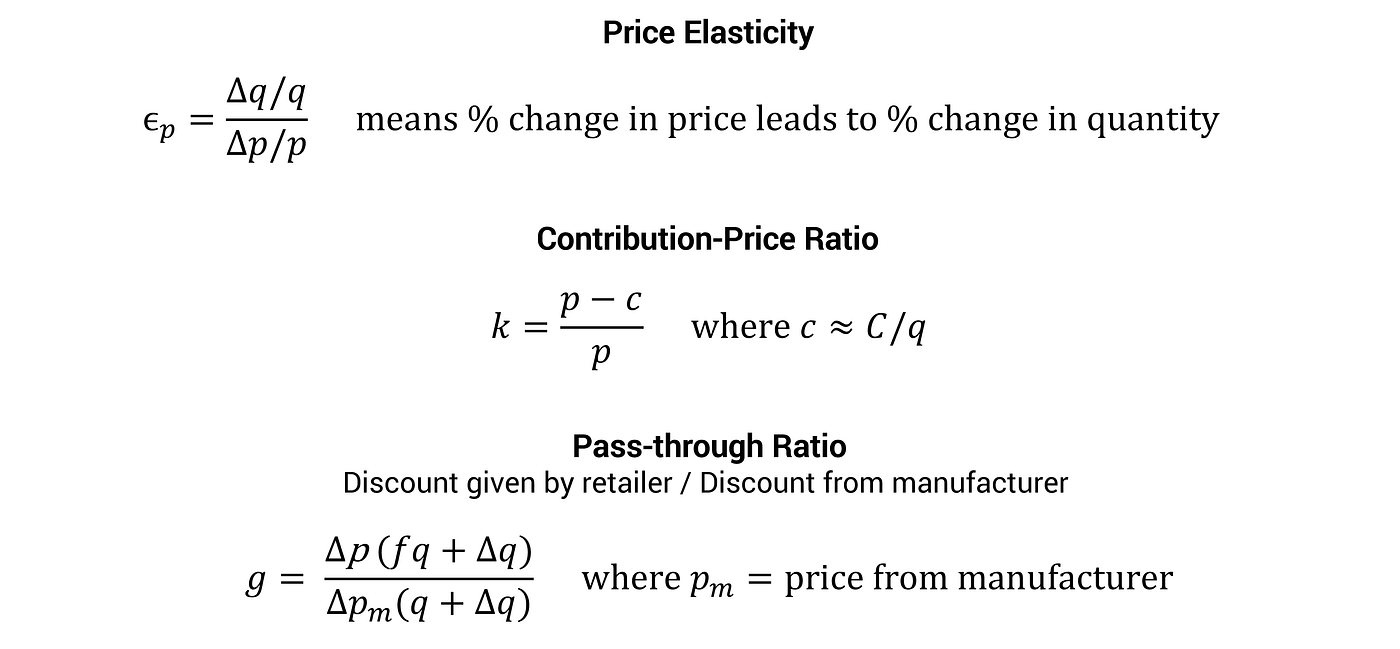Price elasticity ϵₚ is familiar to any reader.² The contribution-price ratio k is intuitive (note that the correct formula is more complex, but this is a good approximation). The pass-through ratio may be less commonly known.
The pass-through ratio is the percentage of a price discount by a manufacturer that is passed on to consumers by retailers. It is by no means a given that if a manufacturer offers a 10% price discount, the retailer also reduces price by 10%.
In affluent countries, modern trade pass through may be 80%. In traditional trade in emerging countries, it may be 40% and in some cases zero.
We also need the fraction of sales that is sold at a discount (f).
With this, the extended equation below is derived. We do not give the proof¹ since our focus is on the implications.
The way to interpret this equation is that for percentage change in price (Δp/p). there is a profit gain proportional to kϵₚ and a profit loss proportional to f/g since the original price is not realized, and the retailer pass through is less than 1.
There is a breakeven price elasticity. A company takes different actions depending on whether the actual price elasticity is above or below this breakeven.
This breakeven elasticity is derived by setting the term in parenthesis to zero. This gives:
If ϵₚ > f/(gk) then the company should reduce price.
We find that it is of critical importance to continuously track four factors:
- Price elasticity ϵₚ. The higher the price elasticity, the more it pays off to discount (if above the breakeven)
- Pass through g. The higher the pass through, the more discounting is suitable.
- Fraction of sales sold at discount f. The less consumers switch to the discounted price away from the original price, the better the discount works.
- Contribution-price ratio k. If contribution is high, companies should be willing to reduce price.³
In inflationary times, these four factors tend to change significantly.
Advertising/Promotion
With less detail, we derive equations for advertising/promo. The advertising/promo elasticity is given by:
The profit formula (here without proof¹) becomes:
For an advertising/promo change ΔA/A there is a profit gain proportional to kϵₐ and a profit loss proportional to the advertising/promo share of sales.
As with price, we find a breakeven elasticity for advertising/promo:
If ϵₐ > (A/S)/k then then the company should increase advertising/promo expenses. If ϵₐ < (A/S)/k then those expenses should be reduced.
The ratios to track for advertising/promo are simpler than for price:
- Advertising/promo elasticity ϵₐ. The higher the elasticity is, the more the company should spend on advertising/promo (if above the breakeven).
- Contribution-price ratio k. If this ratio is high, then the company should spend more on advertising/promo.
- Advertising share of sales A/S. If this ratio is high, the required actual elasticity is high.
Pricing and advertising/promotion together
Finally, we combine the two profit drivers with a realistic example.
Assume:
- Contribution-price ratio k = 0.5
- Fraction of sales sold at discount f = 0.6
- Pass through g = 0.8
- Advertising share of sales A/S = 0.05
This gives a breakeven price elasticity price of 1.5 and a breakeven advertising/promo elasticity of 0.1. Across hundreds of studies, the average price elasticity is around −1.5 and the average advertising elasticity is +0.09 to +0.12 both with large variations around the means.
The graph below summarizes optimal strategies for the example.
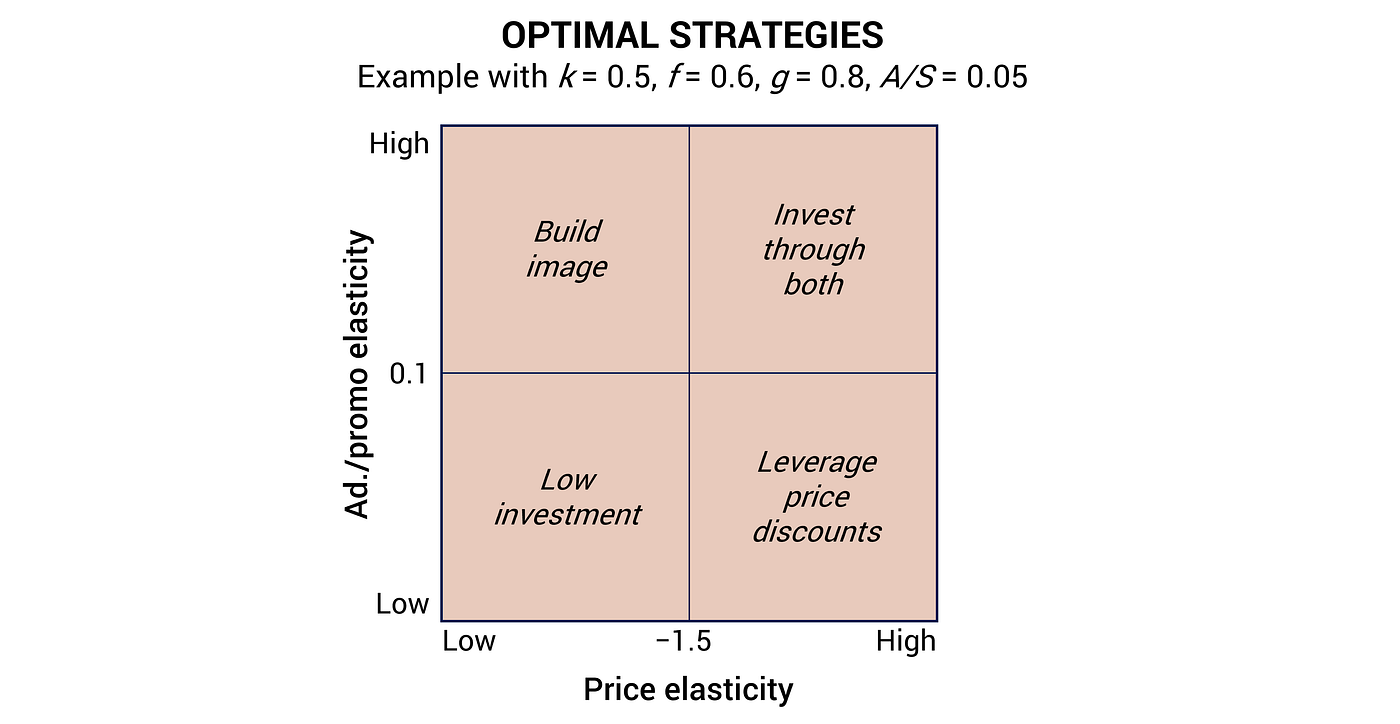
- In the lower left quadrant consumers know what they get and are not swayed by price or advertising/promo. These are typically highly mature niche products.
- The lower right quadrant is typically populated with well-established mass market brands. Consumers know what they get, and advertising does not play a significant role; consumers look for the best deal.
- Upper left quadrant holds luxury goods and new products that require image or informational marketing.
- Differentiated brands are often found in the upper right quadrant, as are seasonal products.
Each quadrant requires its own distinct strategy. For a large consumer goods companies, brands can typically be found in at least three of the quadrants.
CONCLUSION
Updating established beliefs is crucial to any consumer goods company. What used to be in one quadrant may have moved to an entirely new position over the last year.
Beyond this, systematically analyzing the market conditions as described above makes sense any time. The urgency prompted by inflation makes now the time to start.
Many companies have implemented elements of this generalized framework. They may know their elasticities, sometimes they have the contribution figured out.
The goal should be to have all elements implemented in a structured and repeatable fashion across geographies and business units. Few, if any, companies have this.
• • •
To learn more, contact us by filling out this online form.
• • •
¹ R. Sethuraman and G. Tellis: An Analysis of the Tradeoff between Advertising and Price Discounting. Journal of Marketing Research. Vol. 28, №2 (May, 1991), pp. 160–174
² The elasticity symbol ϵ is a lunate epsilon and should be called epsilon. Other ways to denote elasticity are ey/ex (with y and x substituted with variables used), E, el., and possibly more.
³ Note that this is not the EBITDA metric which has nothing to do with contribution (or for that matter, profitability). EBITDA combines some variable and fixed costs and omits many costs. It is perhaps useful in investor presentations as a proxy for cash flow. True variable and fixed costs are best quantified with a regression analysis.